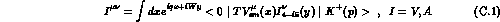
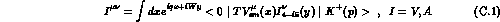
and detail their connection with the matrix element (1.2).
The general decomposition of 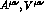 in terms of Lorentz invariant amplitudes reads [9,11] for
in terms of Lorentz invariant amplitudes reads [9,11] for 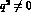
and
where the form factors 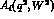 and
and 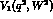 are analytic functions
of
are analytic functions
of  and
and 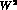 .
. 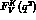 denotes the electromagnetic form factor of
the kaon
denotes the electromagnetic form factor of
the kaon  .
. 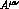 satisfies the Ward identity
satisfies the Ward identity
In the process (1.1) the photon is real. As a consequence of this, only
the two form factors 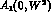 and
and  contribute. We set
contribute. We set
and obtain for the matrix element (1.2)
with
Grouping terms into an IB and a SD piece gives (1.2,1.3).
As a consequence of (C.4), T is
invariant
under the gauge transformation 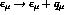 .
.
The amplitudes 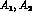 and
and  are related to the
corresponding quantities
are related to the
corresponding quantities 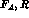 and
and 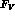 used by the PDG [3] by
used by the PDG [3] by
The last term in (C.2) is omitted in [3]. It
contributes to
processes with a virtual photon, 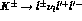 .
.
Finally, the relation to the notation used in [4,5] is